Waves on the surface of a fluid are a common physical phenomenon; mathematical problems of wave theory arise in connection with important practical applications (first of all, calculation of hydrodynamic loads on submerged bodies). Problems are studied that describe in a linear approximation the steady motion of an ideal, heavy fluid with a free surface in the presence of obstacles. Finding uniquely solvable statements is one of the key issues [⤤] , often requiring development of original mathematical methods; for example, consideration of localized (trapped) modes is often reduced to studying point eigenvalues embedded into continuous spectrum.

Main results:

• Conditions for the uniqueness and solvability of a number of problems are found, including the plane problem of fluid flow over a bottom obstacle [⤤] , the problem of forward motion of bodies in a homogeneous [⤤] and two-layer fluid [⤤] , the problem of time-harmonic oscillations of a fluid in the presence of fixed bodies [⤤] , [⤤] and obstacles [⤤] , taking into account surface tension [⤤] , as well as freely floating bodies [⤤] , [⤤] , [⤤] .

• The question of supplementary conditions that turn the Neumann–Kelvin problem into a uniquely solvable one is studied for bodies that either cross the free surface or the interface between two layers of different density [⤤] , [⤤] , [⤤] .

• For the problem of the forward motion of bodies and the problem of fluid oscillations in the presence of bodies, criteria for unique solvability are found. Numerical algorithms are developed and, for the first time, examples of non-uniqueness with completely submerged bodies [⤤] , [⤤] , [⤤] , [⤤] , [⤤] , [⤤] are found (including taking into account surface tension and in a two-layer liquid). The connection of examples of non-uniqueness with anomalously large values of wave resistance is shown. The figure shows a body for which there is an example of non-uniqueness, and a picture of streamlines [⤤].

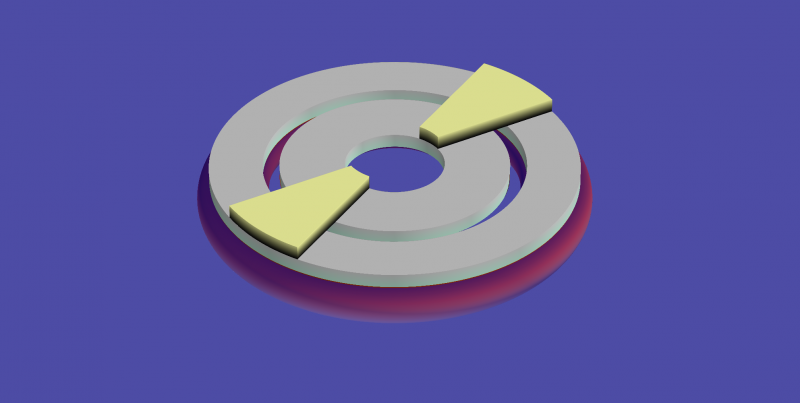
• A number of examples of non-uniqueness for bodies partially submerged in a fluid or crossing the interface are obtained. The inverse procedure is used: for a given velocity potential, a geometry is sought for which it is a solution to the homogeneous problem [⤤] , [⤤]. Significant progress has been made in problems of coupled oscillations of a fluid and freely floating bodies [⤤] , [⤤] and [⤤]. In the constructed examples of mode localization [⤤] , the submerged part of the bodies is axisymmetric, and the bodies are motionless, although they float freely — the figure shows an example of such a structure.

• The problem of fluid oscillations in the presence of partially submerged bodies is considered in the case when the fluid is bounded from above by a floating brash ice. The existence of localized oscillation modes is established, and examples of bodies supporting such modes with finite energy are constructed. On the other hand, we find conditions for the absence of localized modes [⤤] , [⤤].

• The problems of forward motion of bodies and vibrations of bodies in a two-layer fluid are considered. An important feature is a more complex picture of hydrodynamic loads due to the presence of internal waves associated with the phenomenon of "dead water". Question of unique solvability is studied, examples of non-uniqueness are found, properties of solutions are investigated , including hydrodynamic loads and their possible features , [⤤] , [⤤] (the problem of oscillations).

• For the three-dimensional problem of the motion of bodies in a fluid with a free surface (the problem of ship waves) , algorithms for fast calculation of the Green's function and derivatives have been developed. For this purpose, methods have been developed for calculating integrals of rapidly and non-harmonically oscillating functions over a semi-infinite interval [⤤] .

• Eigenmodes of fluid oscillations with a free surface in vertical circular and annular cylindrical containers are studied. The effect of violation of axial symmetry due to the presence of radial baffles going vertically from the free surface to the bottom was analyzed. It is shown that the presence of a baffles results in a significant change in the properties of eigenvalues and eigenfunctions [⤤].